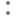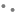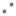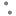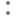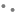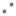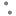求最大的常数 $M$, 使得对任意一个满足如下条 件的无穷实数列 $\left\{a_n\right\}, n=0,1,2, \ldots$, 以及任 意 $n \geq 0$, 均有 $\frac{a_{n+1}}{a_n}>M$ :
(1)$a_0=1, a_1=3$;
(2)$a_0+a_1+\ldots+a_{n-1} \geq 3 a_n-a_{n+1}$.

解:取$a_n=(n+2)2^{n-1}$,则满足全部条件.
此时,$\frac{a_{n+1}}{a_n}=\frac{2(n+3)}{n+2}>M$恒成立.
取$n \to +\infty,M \le 2$
下面证明$M=2$是复合题意的:
令$S_n=a_0+a_1+a_2+\dots+a_n$,则原式化为\[    S_{n-1} \ge 3(S_n-S_{n-1})-(S_{n+1}-S_n)    \]
可得\[    S_{n+1}-2S_n \ge 2(S_n-2S_{n-1})    \]
于是有\[    S_{n}-2S_{n-1} \ge 2^{n-1}(S_1-2S_0)=2^n    \]
容易得到$S_n$递增,$a_n>0$,且\[    a_{n+1}-2a_n \ge a_n-(a_0+a_1+\dots+a_{n-1})=S_n-2S_{n-1}>0    \]
即\[    \frac{a_{n+1}}{a_n}>2    \]
    综上,$M$的最大值是2

已知$a,b,c$是三角形的三边长,求证:
\[    \frac{(a^3+b^3+c^3)^2}{(a^2+b^2)(b^2+c^2)(c^2+a^2)}<2\]

注意到
\begin{align}    &(1+\mi) (a+\mi b) (b+\mi c)(c+\mi a)\\    =&-\left(a^2b+ab^2+b^2c+bc^2+c^2a+ca^2-2abc\right)\\    &+\mi \left(a^2 b-a^2 c-a b^2+a c^2+b^2 c-b c^2\right)\end{align}
取模的平方,可得
\begin{align}    &2(a^2+b^2)(b^2+c^2)(c^2+a^2)\\    =&\left(a^2b+ab^2+b^2c+bc^2+c^2a+ca^2-2abc\right)^2\\    &+\left(a^2 b-a^2 c-a b^2+a c^2+b^2 c-b c^2\right)^2\\    \ge&\left(a^2b+ab^2+b^2c+bc^2+c^2a+ca^2-2abc\right)^2\\    =&[(a+b-c)(b+c-a)(c+a-b)+(a^3+b^3+c^3)]^2\\    >&(a^3+b^3+c^3)^2\end{align}

已知实数$x,y$满足$|x^2+y|-|x+y^2|=2$,则$|x|$的最小值是$\tk$

显然$|x^2+y| =|x+y^2|+2 \ge 2$
若$x^2+y\le -2$,则\[    x+y^2 \ge x+(2+x^2)^2 \ge x+(1+2|x|)^2>x+4|x| \ge 0    \]
从而原式化为$-(x^2+y)-(x+y^2)=2,(x+\frac{1}{2})^2+(y+\frac{1}{2})^2+\frac{3}{2}=0$,矛盾.
所以有$x^2+y \ge 2$
若$|x|<1$,则$y \ge 2-x^2>1$\[    x+y^2>-1+1=0\]
从而原式化为\[    (x^2+y)-(x+y^2)=2,(x-2)(x+1)=y(y-1)    \]
注意到$(x-2)(x+1)<0,y(y-1)>0$,矛盾.
所以有$|x| \ge 1$,注意到$x=-1,y=1$可取等,因此$|x|$的最小值为1

    求出所有的正整数$k$,使得对任意满足不等式    \[        k(ab+bc+ca)>4(a^2+b^2+c^2)        \]        的正数$a,b,c$,一定存在三边长分别为$a,b,c$的三角形

考虑三角形的极端情况$(1,1,2)$,可知$k \le 6$,(否则如果$k>6$,就有$(1,1,2)$满足不等式但是不可以构成三角形)       
而基本不等式可知$k>5$,因此只有$k=6$是可能的   
\[    \frac{6}{5}>\frac{a^2+b^2+c^2}{ab+c(a+b)} \ge \frac{\frac{(a+b)^2}{2}+c^2}{\frac{(a+b)^2}{4}+c(a+b)}            \]
令$\ds t=\frac{a+b}{c}$,得$t^2-6t+5<0,1<t<5$               
这表明总是有$a+b>c$,满足题意

关于$x$的方程$e^x-a \sin x=b\sqrt{x}$有根,求证:$a^2+b^2>\me$

这可真是个老题了,而且各种模拟题早就玩出花了,只是本题增加了一些变式,而且求的不是最值了,而是对最值的估计
这类题最早的出处应该是1973年的IMO(国际数学奥林匹克)第3题:

    3. 设 $a, b$ 是可使方程    \[    x^{4}+a x^{3}+b x^{2}+a x+1=0    \]    至少有一实根的实数, 对于所有这样的数偶 $(a, b)$, 求 $a^{2}+b^{2}$ 的最小值.

    当年作为国际比赛的压轴题,而今已经沦为而今竞赛教材的经典问题,一般放在一试里.
    显然$x \ne 0$,则可变形为$(x^2+\frac{1}{x^2})+a(x+\frac{1}{x})+b=0$,令$u=x+\frac{1}{x}$则有    \[        u^2-2+au+b=0,u^2 \ge 4        \]        由柯西不等式    \[        (u^2-2)^2=(au+b)^2 \le (a^2+b^2)(u^2+1)        \]
    从而    \[        a^2+b^2 \ge (u^2+1)+\frac{9}{u^2+1}-6 \ge \frac{4}{5}        \]        且$a=\frac{4}{5},b=-\frac{2}{5}$时方程有根$x=-1$,故可以取到等号.

    本题作为这类题的题源,经过各种变迁,如果将其中一些函数换为指数等特殊函数,则可以与导数联系起来,在各地模拟题或者网上自编题中已经出现了一些.凡是学过竞赛的同学都有入手的方向,但是直接进入高考试卷仍然是十分激进的.

    对于本题的解答:
    这个根$x$肯定是正的,由柯西不等式以及$\sin x<x,x>0$    \[        \me^x=a\sin x+b\sqrt{x} \le \sqrt{a^2+b^2}\sqrt{\sin^2 x+x} < \sqrt{a^2+b^2}\sqrt{x^2+x}        \]        若$0<x\le 1$,使用不等式$\me^x \ge \me x$    \[        a^2+b^2>\frac{\me^{2x}}{x^2+x} \ge \frac{\me \cdot 2x}{x^2+x}=\me \cdot \frac{2}{x+1} \ge \me        \]        若$x>1$,再次使用不等式$\me^x \ge \me x$,以及$\frac{x}{x+1}>\frac{1}{2}$    \[        a^2+b^2>\frac{\me^{2x}}{x^2+x} \ge \frac{(\me x)^2}{x^2+x}=\me^2 \frac{x}{x+1}>\frac{\me^2}{2}>\me    \]
    这样就证明了原题.

参考答案简直丑陋.给个简单解法.

14. 设数列 $\left\{a_{n}\right\}$ 满足 $\ds a_{1}>0, a_{n+1}=a_{n}+\frac{n}{a_{n}}(n \geq 1)$, 证明
(1).数列 $\left\{a_{n}-n\right\}(n \geq 2)$ 为单调递减;
(2).存在一个常数 $c$ 使得 $\ds \sum_{k=1}^{n} \frac{a_{k}-k}{k+1} \leq c, n \geq 2$ 。

(1). 归纳证$a_n \ge n,\forall n \ge 2$,然后可得$\ds a_{n+1}-(n+1)=(1-\frac{1}{a_n})(a_n-n)<a_n-n$       

(2).由(1)可知,当$n \ge 2$时$a_{n}-n\le a_2-2$                 
取$\lambda=\max\{1,a_2-2\}$,则$a_n \le n+(a_2-2)\le \lambda (n+1)$        \[            (a_k-k)-(a_{k+1}-(k+1))=\frac{a_k-k}{a_k} \ge \frac{a_k-k}{\lambda (k+1)}            \]
        累加可得        \[            \sum_{k=2}^n \frac{a_k-k}{k+1} \le \lambda[(a_2-2)-(a_{n+1}-(n+1))] \le \lambda (a_2-2)        \]
        从而        \[            \sum_{k=1}^{n} \frac{a_{k}-k}{k+1} \le \frac{a_1-1}{2}+\lambda (a_2-2)        \]
        因此,取$\ds c=\frac{a_1-1}{2}+\lambda (a_2-2)$即可 

参考答案如下:

锐角$\triangle ABC$中,求$\tan A+8\tan B+13 \tan C$的最小值

设$x=\tan A>0,y=\tan B>0,z=\tan C>0$,则$xyz=x+y+z$
\begin{align}    x+8y+13z&=\frac{1}{z}[(xz-1)+8(yz-1)]+\frac{9}{z}+13z\\    &\ge \frac{1}{z} \cdot 2\sqrt{8(xz-1)(yz-1)}+\frac{9}{z}+13z\\    &= \frac{1}{z} \cdot 2\sqrt{8[z(xyz-x-y)+1]}+\frac{9}{z}+13z\\    &= \frac{4}{z} \sqrt{2} \cdot \sqrt{z^2+1}+\frac{9}{z}+13z\\    & \ge \frac{4}{z}(z+1)+\frac{9}{z}+13z\\    & =4+13(z+\frac{1}{z}) \ge 30\end{align}

当$\ds z=1,y=\frac{3}{2},x=5$取等
故$\tan A+8\tan B+13 \tan C$的最小值是30

另解:
齐次化用取等凑30元均值

注意到

\begin{align}    x+8y+13z=\frac{x}{5} \times 5+&\frac{2y}{3} \times 12+z \times 13\\    &\ge 30\sqrt[30]{(\frac{x}{5})^5 (\frac{2y}{3})^{12} z^{13}}\\    x+y+z=\frac{x}{10} \times 10+&\frac{y}{3} \times 3+\frac{z}{2} \times 2\\    &\ge 15 \sqrt[15]{(\frac{x}{10})^{10} (\frac{y}{3})^3 (\frac{z}{2})^2}\\    (x+8y+13z)^2 (x+y+z) \\    \ge 30^2 \sqrt[15]{(\frac{x}{5})^5 (\frac{2y}{3})^{12} z^{13}} &\times 15 \sqrt[15]{(\frac{x}{10})^{10} (\frac{y}{3})^3 (\frac{z}{2})^2}=30^2 xyz\end{align}

结合$xyz=x+y+z$,可得$x+8y+13z \ge 30$

注:换系数会导致题目不可解,变为高次方程的根

对于$a,b,c \in \mR$,求$\ds f=\frac{\sqrt{(x^2+3)(y^2+3)(z^2+3)}-2x}{y+z}$的最小值

由Aczel不等式,\begin{align}    f&=\frac{\sqrt{(x^2+3)(y^2+3)(z^2+3)}-2x}{y+z}\\    &=\frac{\sqrt{x^2+3} \cdot \sqrt{(y^2+3)(z^2+3)}-x \cdot 2}{y+z}\\    &\ge \frac{\sqrt{3} \cdot \sqrt{(y^2+3)(z^2+3)-4}}{y+z}\\    &=\sqrt{3}\sqrt{\frac{(y^2 z^2+5)+3(y^2+z^2)}{2yz+(y^2+z^2)}}\\    & \ge \sqrt{3}\sqrt{\frac{2\sqrt{5}yz+3(y^2+z^2)}{2yz+(y^2+z^2)}},(s=\frac{y^2+z^2}{2yz} \ge 1)\\    & =\sqrt{3}\sqrt{\frac{\sqrt{5}+3s}{1+s}}\\    & \ge \sqrt{3}\sqrt{\frac{3+\sqrt{5}}{2}}=\frac{\sqrt{3}+\sqrt{15}}{2}\end{align}
取等时$y=z=\sqrt[4]{5},x=…$

本题是一个稍有难度的不等式

已知$x_{i} \in \mR(1 \leq i \leq 8)$ 的和为 8, 其中最大的数不超过最小的数的 3 倍, 则 $\ds \sum_{i=1}^{8} x_{i}^{2}$ 的最大值为

不妨设$m=\min x_i,M=\max x_i$,则$m \le x_i \le M, \forall i=1,2,\dots,8$,且$0<m \le 1 \le M \le 3m$
故$(x_i-m)(x_i-M) \le 0,x_i^2 \le (M+m) x_i-Mm$
对上式求和并考虑到$m \le 1$,有
\begin{gather}    \sum_{i=1}^8 x_i^2 \le (M+m) \sum_{i=1}^8 x_i-8Mm=8(M+m-Mm)\\    =8M(1-m)+8m \le 8 \cdot 3m (1-m)+8m    \le \frac{32}{3}\end{gather}
当$\ds x_1=x_2=\dots=x_6=\frac{2}{3},x_7=x_8=2$时可取等
故 $\ds \sum_{i=1}^{8} x_{i}^{2}$ 的最大值为$\ds \frac{32}{3}$

关于这份题目我只看到网上写的个别题的解答,但这个题他做错了

对于$\max$函数,我们常用的是\[\max(a,b) \ge a,\max(a,b) \ge b\]
而实际上我们还可以使用它的加权形式:\[\forall t \in [0,1],\max(a,b) \ge ta+(1-t)b\]

1.$a,b>0$,求$\ds h=\max(a,\frac{1}{b})+\max(b,\frac{2}{a})$的最小值

引入参数$x,y \in [0,1]$
\[h \ge xa+(1-x)\frac{1}{b}+y\frac{2}{a}+(1-y)b \ge 2\sqrt{2xy}+2\sqrt{(1-x)(1-y)}=f(x,y)\]
由于此式是恒成立,所以\[h \ge \max f(x,y)\]
而\[f(x,y)=2(\sqrt{2x}\sqrt{y}+\sqrt{1-x}\sqrt{1-y}) \le 2\sqrt{2x+1-x}\sqrt{y+1-y}=2\sqrt{x+1} \le 2\sqrt{2}\]
且于$x=1,y=1$取等

故可得$h \ge 2\sqrt{2}$.且根据取等的$x,y$可以写出\[h \ge a+\frac{2}{a} \ge 2\sqrt{2}\]

2.$a,b,c>0$,求$\ds h=\max(a,\frac{1}{b})+\max(b,\frac{2}{c})+\max(c,\frac{3}{a})$的最小值

同理,我们引入$x,y,z \in [0,1]
$\begin{gather*}h \ge xa+(1-x)\frac{1}{b}+yb+(1-y)\frac{2}{c}+zc+(1-z)\frac{3}{a}\\\ge 2\sqrt{3(1-z)x}+2\sqrt{(1-x)y}+2\sqrt{2(1-y)z}=f\\\frac{f}{2}=\sqrt{3x}\sqrt{1-z}+\sqrt{2(1-y)}\sqrt{z}+\sqrt{(1-x)y}\\\le \sqrt{3x+2(1-y)}\sqrt{1-z+z}+\sqrt{(1-x)y}\\=\sqrt{x+\frac{2(1-y)}{3}}\sqrt{3}+\sqrt{1-x}\sqrt{y}\\\le \sqrt{1+\frac{2(1-y)}{3}}\sqrt{3+y}=\sqrt{\frac{1}{3}(15-y-2y^2)} \le \sqrt{5}\\f \le 2\sqrt{5}\end{gather*}
取等时\[y=0,x=1,z=\frac{2}{5}\]
知道取等以后就可以用下面这种看起来很厉害的办法写过程了
\begin{gather*}h=\max(a,\frac{1}{b})+\max(b,\frac{2}{c})+\max(c,\frac{3}{a})\\\ge a+\frac{2}{c}+(\frac{2}{5}c+\frac{3}{5}\frac{3}{a})=a+\frac{9}{5a}+\frac{2}{c}+\frac{2c}{5}\\\ge 2\sqrt{\frac{9}5}+2\sqrt{\frac{4}{5}}=2\sqrt{5}\end{gather*}
只需要举出一例保证可以取到即可

很明显,如果最后关于$y$的二次函数取最值不在端点,整个式子就会更加复杂.按照这种思路,就可以自己命制新的问题了

3.$a,b,c>0$,求$\ds h=\max(a,\frac{4}{b})+\max(b,\frac{2}{c})+\max(c,\frac{3}{a})$的最小值

\begin{gather*}h=\max(a,\frac{4}{b})+\max(b,\frac{2}{c})+\max(c,\frac{3}{a})\\\ge \frac{5}{12}a+\frac{7}{12}\frac{4}{b}+\frac{7}{8}b+\frac{1}{8}\frac{2}{c}+\frac{1}{6}c+\frac{5}{6}\frac{3}{a}\\=5(\frac{a}{12}+\frac{1}{2a})+7(\frac{b}{8}+\frac{1}{3b})+(\frac{c}{6}+\frac{1}{4c})\\\ge 10\sqrt{\frac{1}{24}}+14\sqrt{\frac{1}{24}}+2\sqrt{\frac{1}{24}}=\frac{13\sqrt{6}}{6}\end{gather*}
当且仅当$\ds a=\sqrt{6},b=\frac{2\sqrt{6}}{3},c=\frac{\sqrt{6}}{2}$取等

其实就是三个$\max$里面的东西同时相等\[a=\frac{4}{b},b=\frac{2}{c},c=\frac{3}{a}\]
而后再根据这个取等凑系数.这句话很重要,不再举例.